About me and why I created this physics website.

Velocity Problems

On this page I put together a collection of velocity problems to help you understand velocity better. The required equations and background reading to solve these problems is given on the kinematics page.

Problem # 1

A car travels at uniform velocity a distance of 100 m in 4 seconds. What is the velocity of the car? (Answer: 25 m/s)


Problem # 2

A sailboat is traveling north at 10 km/h, relative to the water. The water is flowing north at 5 km/h. What is the velocity of the boat relative to ground? (Answer: 15 km/h)


Problem # 3

A sailboat is traveling north at 10 km/h, relative to the water. The water is flowing west at 5 km/h, parallel to the shore. What is the velocity of the sailboat relative to ground, and what is the angle of travel that the sailboat makes with respect to the shore? (Answer: 11.18 km/h, 63.43 degrees or 26.57 degrees)


Problem # 4

In problem # 3, a woman is running at 4 km/h along the shore in the opposite direction to the water's flow. What is the velocity of the sailboat relative to the woman and what angle of travel does the sailboat make with respect to her? (Answer: 13.45 km/h, 48.01 degrees or 41.99 degrees)


Problem # 5

If a sprinter runs 100 m in 10 seconds, what is his average velocity? (Answer: 10 m/s)


Problem # 6

The world record for the men's marathon is 2:03:38. If the distance is 42.195 km, what is the average velocity during the run? (Answer: 5.69 m/s)


Problem # 7

A plane needs to reach a velocity of 300 km/h relative to the air in order to take off. If there is a wind blowing at 80 km/h in the direction opposite to take off, what velocity must the plane reach relative to ground in order to take off. (Answer: 220 km/h)


Problem # 8



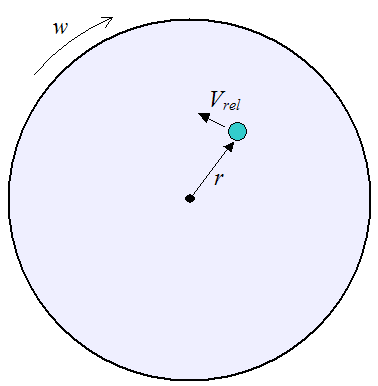
A child on a horizontal merry-go-round gives an initial velocity V_rel to a ball. Find the initial direction and velocity V_rel of the ball relative to the merry-go-round so that, relative to the child, the ball goes around in a perfect circle as he’s sitting on the merry-go-round. Assume there is no friction between merry-go-round and ball.

The merry-go-round is rotating at a constant angular velocity of w radians/second, and the ball is released at a radius r from the center of the merry-go-round.

Solution


Problem # 9

The seals around an engine piston are designed for a maximum velocity of 5 m/s relative to the piston wall. If the piston oscillates sinusoidally with a stroke length of 20 cm, what is the maximum engine RPM?

Hint and answer

The position of the piston can be expressed as P = (20/2)sin(2πft), where f is the engine frequency in Hz, and t is time. Differentiate this expression with respect to time to give the velocity of the piston. We have V = 20πfcos(2πft). The maximum velocity is equal to 20πf which is equal to 500 cm/s, as stated in the problem. Solve for f: f = 25/π Hz, which equals 25×60/π = 477.5 RPM.



Return to Kinematics page


Return to Real World Physics Problems home page